Difference between revisions of "Wave mechanics"
Jump to navigation
Jump to search
| Line 14: | Line 14: | ||
w = 2πf = 2π/T where T = period | w = 2πf = 2π/T where T = period | ||
| − | So if given the amplitude, wavelength and frequency of a particle that was behaving in a sine fashion, its position could be calculated at a particular time on the t axis using this equation. It would also give the particle position as a function of time at a particular distance on the x axis. Both plots | + | So if given the amplitude, wavelength and frequency of a particle that was behaving in a sine fashion, its position could be calculated at a particular time on the t axis using this equation. It would also give the particle position as a function of time at a particular distance on the x axis. Both plots y vs. x and y vs. t would be sine plots. |
Revision as of 12:15, 5 May 2020
Introduction
Basic Equation
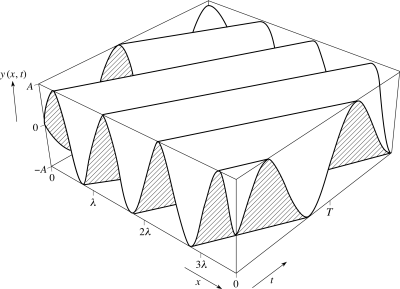
The basic equation of wave mechanics is a function that describes the position of a particle as a function of time and displacement, where the function is wavelike, such as sine or cosine:
y(x,t) = Ψ = Asin(kx - ωt)
A = amplitude
k = 2π/λ where λ = wavelength
w = 2πf = 2π/T where T = period
So if given the amplitude, wavelength and frequency of a particle that was behaving in a sine fashion, its position could be calculated at a particular time on the t axis using this equation. It would also give the particle position as a function of time at a particular distance on the x axis. Both plots y vs. x and y vs. t would be sine plots.